In lower plot, a schematic representation of a periodic array of double-well potential along the x a-xis with depth V_0. That "superlattice" potential is created by superimposing two optical lattice potentials differing in spatial period by a factor of two, 325 nm and 765 nm in the experiment by the group of prof. I. Bloch at the University of Mainz, Germany.

Schematic representation of the superexchange spin interactions between ultracold atoms located in minima of a double-well potential. (Courtesy of Prof. I. Bloch, University of Mainz, Germany)

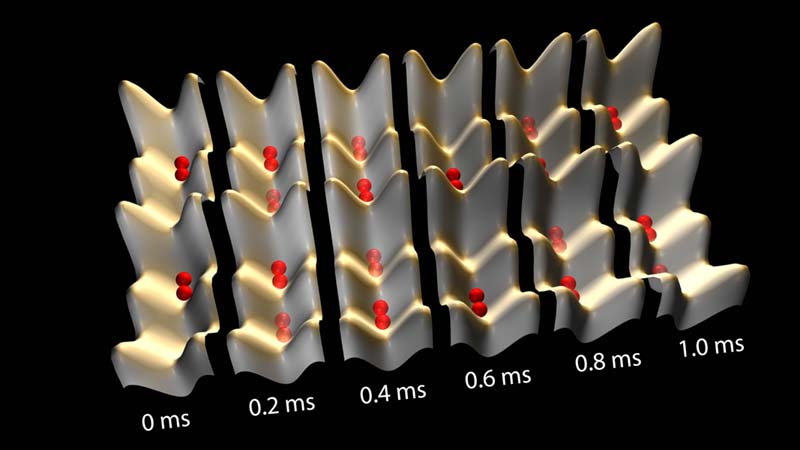
Time evolution of the correlated tunnelling of two interacting ultracold atoms through a barrier in a double-well potential. Two separate double-wells are, upper and lower part of each plot, are presented. At times 0.2, 0.4, 0.8 and 1 ms the two-atom wavefunction corresponds to a superposition between two neighbouring wells.(Courtesy of Prof. I. Bloch, University of Mainz, Germany)

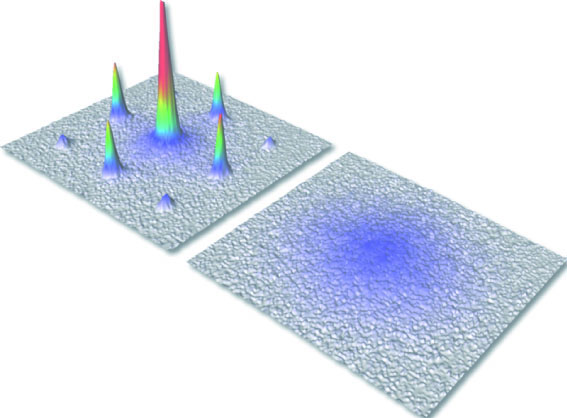
High contrast interference patterns observed in two dimensions for the atoms released from the optical lattice.
Left image: interference pattern produced in the superfluid regime where the delocalized condensate corresponds to a macroscopic wavefunction distributed over the whole lattice.
Right image: interference pattern produced in the quantum phase transition to the Mott-Insulator regime. No phase coherence exists for the wavefunctions associated to atoms localized in the minima of the optical lattice. (Courtesy of Prof. I. Bloch, University of Mainz, Germany)

The distribution of the atomic momenta is detected by applying a Brillouin zone maping, i.e. observing the population of the corresponding Brillouin zones.(Courtesy of Prof. I. Bloch, University of Mainz, Germany)

Brillouin zone mapping combined with a Stern-Gerlach filter can be used to observe spin dynamics for atoms located in the double-wells of the optical superlattice. (Courtesy of Prof. I. Bloch, University of Mainz, Germany)

Left image: The absorption image of a Mott insulating atomic cloud is just a simple gaussian and does not contain any information on the many particle state in the lattice.
Right image: in the same regime, the noise is correlated at distances depending on the initial periodic separation of the particles in the trap. (Courtesy of Prof. I. Bloch, University of Mainz, Germany)

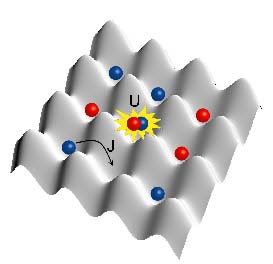
Model for model ultracold atoms trapped by an optical lattice. Atoms in the lowest band of an optical lattice can tunnel between lattice sites with a tunnelling rate J. Two atoms with localized at the same lattice site have an interaction energy U, which can be likewise positive (repulsive interaction) or negative (attractive interaction). The interplay between U and J determine the physics of the Hubbard Hamiltonian for bosons or fermions. (Courtesy of Prof. T. Esslinger, ETH, Zurich, Switzerland)

Photo of the key components for the PISA experiment, with the quartz cell at the center of the magnetic coils producing the magnetic trap. The coloured lines denote the propagation directions of the laser beams interacting with the condensate: MOT beams in red, dipolar trap beams in green, optical lattice beams in orange, Rydberg state excitation beam in blue, imaging laser beam in light blue.

A Bose-Einstein condensate held by an optical lattice (top blob) "leaks out" due to the Earth's gravitational force. The matter waves escaping from the lattice interfere with one another, resulting in the blobs visible below the "parent" condensate.