
Optical Lattices and
Quantum Information
|

 Community Research |
 Specific Targeted Research Projects Sixth Framework Programme |


A brief introduction to quantum computing and the OLAQUI project
|
The OLAQUI project aims to combine two modern scientific concepts – optical lattices and quantum information. In this context, “optical lattices” are the tool and “quantum information” is the desired objective. “Quantum information” is a buzzword that has been around for more than a decade now, and in reality it says something rather simple: quantum physics can help to improve information technology, and in particular it can potentially make computers a lot more powerful than would be possible with conventional technology. How so?
How quantum computing worksWhile the details of how quantum computing works are quite intricate, the underlying concept is straightforward. We just need to remember how an ordinary computer works, forgetting for a moment about operating systems, hard drives and all the rest. At the most basic level, all that a computer does is to take some input and to return an output. The input can in principle be anything, for example a text, pictures, even speech, but the computer will always convert these into numbers and process them using basic logical operations. The numbers themselves are represented in the binary system as sequences of “0”s and “1”s (the number “5” in the binary system reads “101”, for instance) and then processed using so-called “logic gates”. One such gate is the NOT gate that takes one bit (i.e. one item of the binary string) as an input and gives the “negation” of it as an output: “0” if the input was “1”, and “1” if the input was “0”. Other logic gates use two input bits and return one or two output bits. Finally, lots of these logic gates stringed together produce the desired computation – simple addition or multiplication or complex tasks like word processing (see Fig. 1). So much for “classical computing”, as we now call it. “Quantum computing” adds an important element to that picture – it replaces the classical “bit” by the quantum-bit or “qubit”. A “qubit” obeys the quantum-mechanical superposition principle and can, therefore, not only exist in the states “0” and “1”, but also in superposition states that are somewhere in between and carry a degree of “0”-ness and “1”-ness in them. We’ll explain this better in a moment, but even with this simple explanation one can appreciate the potential impact of these qubits: if one no longer uses “0”s and “1”s as the input of a computer, but a string of qubits each representing “0” and “1” at the same time, as it were, then that’s as though with a single input one could process all the possible combinations of “0”s and “1”s in that string – that is, all possible numbers – in one single run of the computer. In other words, rather than processing the numbers 1, 2, 3, 4 etc. one after the other, by using qubits they can be processed in one go. This principle makes it possible to devise quantum algorithms – computer programmes for quantum computers – that can solve certain types of problems much, much faster than any present or future supercomputer based on classical physics and bits. For example, breaking a large number down into its prime factors takes a very long time, and this time increases extremely fast with the length of the number. Another algorithm that might be useful in practical applications deals with searching large databases, and again using quantum computers could result in faster search routines. 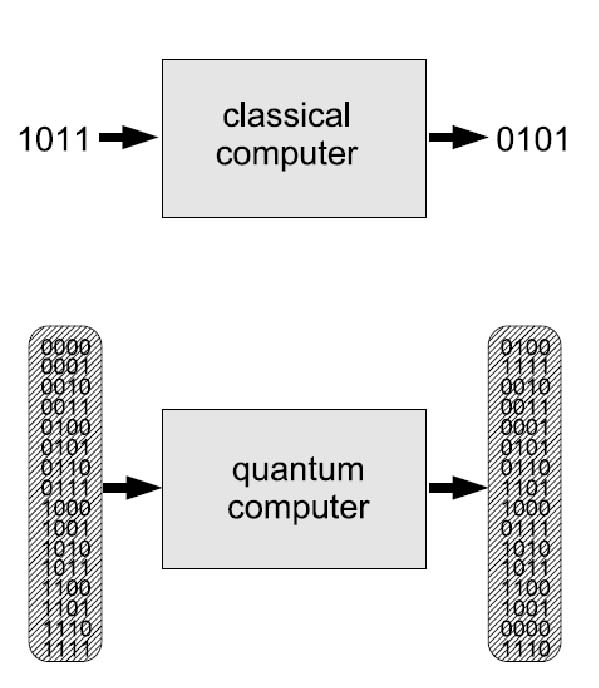
Fig. 1 - Classical computer vs. quantum computer.
Why it is difficult to make a quantum computerIf the last few sentences contained a number of if’s and might’s, that’s because although quantum computers exist on paper and in the minds of theoretical physicists and mathematicians, so far no-one has been able to build a practically usable machine that uses qubits instead of bits. In a nutshell, the reason for this is the following: it is very hard to make and control “qubits”, and even harder to do so with lots of qubits at the same time. “Making” a qubit here means finding a physical object that behaves in a quantum way, so to speak, and therefore can exist in the superposition states needed for quantum computation. An obvious counterexample: an electrical switch wouldn’t do as a qubit. Although such a switch could very well represent a classical bit through its states “switch open” and “switch closed” (and a light bulb attached to it indicating that state), it could not exist in those two states simultaneously. Again, that seems just as obvious as the fact that a person might be either in front of a closed door or behind it, but not in both places at the same time. In the quantum world of atoms and elementary particles, however, this happens all the time. The spin of an atom (that is, the direction of its magnetization) can point either up or down, like a compass needle – or it can be in a superposition of these two states, pointing in both directions at the same time, so to speak. Only when one measures this direction does the atom make up its mind and “collapse” into one of the spin directions. There are countless other examples for such superposition states, and it is only when going up to larger, visible scales that the underlying “quantumness” of the world gets watered down and we end up seeing “classical” – that is, in our experience “normal” – behaviour. So, if one wants qubits, one needs to use atoms or similarly small particles that behave according to the laws of quantum mechanics. In the past decade many more or less suitable candidates for the role of the “qubit” in quantum computing have been proposed. Charged atoms, also called ions, seemed for a long time to be the best choice: being as small as an atom, they show quantum behaviour, and their electrical charge allows one to hold them in place and manipulate them using electric fields. This last point is important because one cannot just put a quantum particle in a box or glue it to some support – any contact with another object will inevitably interfere with is quantum properties and render quantum computation impossible. And even if one uses electric field to hold ions in place, one still has to deal with other problems, such as electrical noise that can lead to an effect called “decoherence”: a qubit that starts out, as it should, in a superposition state of “0” and “1” ends up being in just one of the two states – and hence useless for quantum computing.
Doing quantum computation with neutral atomsThis is where OLAQUI comes in, proposing a different approach to creating and controlling qubits. Rather than charged particles we use neutral atoms, which immediately eliminates a number of problems including the sensitivity to electric fields. The atoms are held in place by so-called optical lattices, created by laser beams. The laser beams are made to intersect, leading to a phenomenon called interference, much like ripples on a lake crossing each other and creating fascinating patterns. The patterns created by the interfering lasers look like three-dimensional egg cartons or, more poetically, crystals made of light. If one chooses the right kind of atom and the right colour of the laser beams, the laser light will exert a force on the atoms and pull them to the regularly arranged bright spots inside the light crystal (see Fig. 2). In order for them to stay there, the atoms have to be extremely called and practically motionless, which makes it necessary to use laser cooling methods before putting them into an optical lattice (at those low temperatures - just a few millionths of a degree above absolute zero - the atoms actually coalesce into a Bose-Einstein condensate). Once that has been achieved, one has an orderly array of cold and, therefore, highly “quantum” atoms – an ideal starting point for doing quantum computation! In fact, one of the main advantages of the approach proposed by the OLAQUI project compared to, for instance, trapped ions, is the fact that capturing millions of atoms inside the light crystal is no harder than it is to trap just a few. 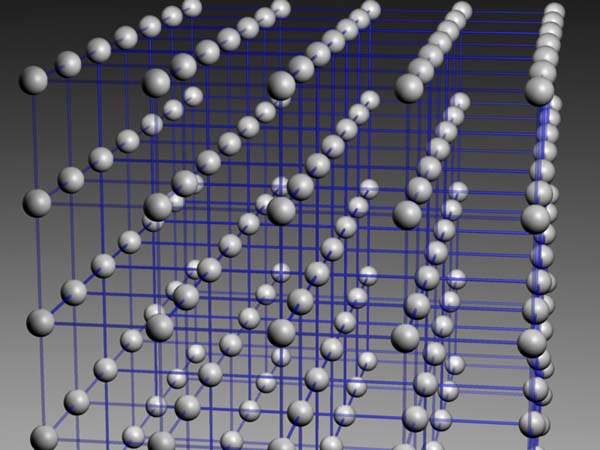
Fig. 2 - A three-dimensional optical lattice. Of course, things are not quite that simple. In fact, putting cold atoms into an optical lattice will not necessarily lead to an orderly filling of the egg carton – there will be lots of empty sites, and some will be occupied by more than one atom. This is not good, so one needs to add an extra step and create a so-called Mott insulator in which, this time around, the atoms do actually occupy all the sites one by one, without leaving gaps. Even if we now have an orderly array of potential qubits – we also call this a “quantum register” – we still have to find a way to manipulate the atoms and to make to neighbouring atoms interact with each other. The last point is important because a quantum computation consists of a series of logic gates applied to one or more qubits. In order to make a quantum logic gate with two qubits, these qubits somehow have to “feel” each other. In optical lattices, this can be achieved by creating overlapping lattices for different quantum states of the atoms and then shifting these lattices with respect to each other. While the techniques for preparing atoms in an optical lattice and making them collide in a controlled way were pioneered by members of the OLAQUI-consortium, a lot remains to be done. Theorists and experimentalists in OLAQUI work together to improve on existing techniques and develop new ones in order to initialize a quantum register of atoms in a lattice and to process the input through quantum gates involving more than one atom. This will be an important step towards building a quantum computer with a large number of qubits. |
